Improvements to the Up Peak Round Trip Time Calculation
Dr Richard D Peters, Peters Research Ltd
This paper was published in the International Journal of Elevator Engineers. Volume 3 No 1 (2000). It is reproduced with permission from The International Association of Elevator Engineers. This web version © Peters Research Ltd 2009.
Abstract
Most lift designs are based on up peak calculations. The up peak is not always the most appropriate choice of peak period for the analysis. Nevertheless, the up peak calculation is important as an industry standard benchmark calculation, and a good starting point for assessing the handling capacity of a lift system. Improvements to the “standard” up peak calculation have been proposed. These include: (a) Introduction of formulae for the calculation of flight times. These formulae can be used for any travel distance and lift dynamics. (b) Formulation of adjustments made for lifts which do not reach rated speed in a single floor jump. (c) Introduction of an express zone term into the Round Trip Time equation.
LIST OF SYMBOLS
| a | acceleration (m/s2) |
| CC | car (rated) capacity (persons) |
| CF | capacity factor (%) |
| df | average inter-floor height (m) |
| dfn | height floor n (m) |
| dH | distance to reach reversal floor H excluding express zone (m) |
| dX | total height of unserved floors in express zone (m) |
| H | average highest reversal floor |
| j | jerk (m/s3) |
| L | number of lifts |
| N | number of floors above main terminal |
| S | average number of stops |
| T | cycle time (s) |
| ta | advanced door opening time (s) |
| tc | door closing time (s) |
| tfd(d) | flight time flor travel distance d (s) |
| tfl | single floor flight time (s) |
| tl | passenger loading time per person (s) |
| t0 | door opening time (s) |
| tp | average passenger transfer time (s) |
| tu | passenger unloading time per person (s) |
| tv | time to travel between two adjacent floors at rated speed (s) |
| ts | time consumed when making a stop (s) |
| tstart | allowance for motor start delay (s) |
| P | average number of passengers in car |
| %POP | 5 minute up peak handling capacity (% population) |
| RTT | rount trip time (s) |
| Ueff | effective building population (persons) |
| Ui | population of floor i (persons) |
| UPPHC | up peak handling capacity (persons/5min) |
| UPPINT | average up peak interval (s) |
| v | contract (rated) speed (m/s) |
Introduction
Most lift designs are based on up peak calculations. The up peak is not always the most appropriate choice of peak period for the analysis. Nevertheless, the up peak calculation is important as an industry standard benchmark calculation, and a good starting point for assessing the handling capacity of a lift system.
The up peak lift calculation is based on estimating the time taken for a lift to make a single “round trip” of the building. The calculation assumes people load the lift at the lowest floor, and get dropped off as the lift stops off at upper floors. The lift then expresses back to the ground floor (some designers include an allowance for additional stops made by the lift on its return journey). The Round Trip Time (RTT) is calculated for a single lift, so results for two of more lifts are extrapolated accordingly.
The up peak calculation has evolved over a number of years. Jones[1] determined results for the probable number of stops made by the elevator during its round trip. Schroeder[2] determined formulae for highest reversal floor. Barney and dos Santos[3] formalised the method with formulae that are now generally accepted by the Lift Industry. A summary of these formulae follow.
Up peak formulae
The average number of passengers assumed to load into a car during up peak traffic is
 (1)
(1)
The effective building population of the buildings is
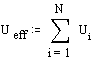 (2)
(2)
The average highest reversal floor is
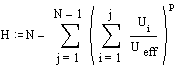 (3)
(3)
The average number of stops made by the lift during its round trip is
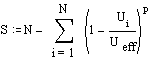 (4)
(4)
The average time taken for a single person to load or unload the lift is
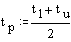 (5)
(5)
The time taken for the lift to travel between two adjacent floors at rated speed is
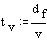 (6)
(6)
The single floor flight time, tf1 is taken from Table 1 [3].
| Contract Speed (m/s) | Acceleration (m/s2) | Single Floor Flight Time, 3.3m floor height(s) |
| 1.00 | 0.4-0.7 | 7.0 |
| 1.50 | 0.7-0.8 | 6.0 |
| 2.50 | 0.8-0.9 | 4.8 |
| 3.50 | 1.0 | 3.7-4.0 |
| 5.00 | 1.2-1.5 | 3.7-4.0 |
Table 1 Typical flight times
The cycle time is the time to travel a single floor, and open/close the doors
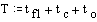 (7)
(7)
So the delay or “time consumed” by making a single stop is
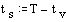 (8)
(8)
The RTT is the time taken for the travel to/from the highest reversal floor at contract speed, plus the delay for each stop, plus the time for the passengers to load/unload. Thus,
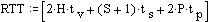 (9)
(9)
Some designers add 5-10% to the RTT for “losses” associated with controller inefficiencies, passengers holding the doors, and so on.
The up peak interval is calculated by dividing the round trip time by the number of lifts.
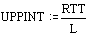 (10)
(10)
The interval is the average time between successive lift arrivals at the main terminal floor. It is not the average waiting time, which Strakosch states is about 55 to 60% of the interval, dependant on the control system[4].
The up peak handling capacity is the number of passengers transported in a five minute period. This is calculated as
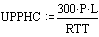 (11)
(11)
The handling capacity, expressed as a percentage of the building population transported in five minutes is
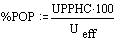 (12)
(12)
Improvements to Calculation
Flight time calculation
Determining flight time from Table 1 is limited as the inter-floor heights are assumed to be 3.3 m, and only “standard” speeds and accelerations are considered. The author’s research in ideal lift kinematics [5][6] has yielded general formulae to determine flight time for any travel distance and lift dynamics.
if 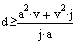 then
then 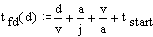 (13)
(13)
if  then
then 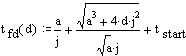 (14)
(14)
if 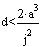 then
then 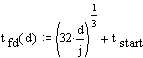 (15)
(15)
These formulae are consistent with results provided by Molz [7], but are in a simpler form.
Applying the function, the single floor flight time is
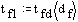 (16)
(16)
Lifts not reaching full speed in single floor jump and non-equal inter-floor heights
The conventional RTT equations assume that the lift reaches rated speed in the distance of a single floor jump; and that there are no irregularities in floor heights. This is not always the case, and CIBSE Guide D [8] proposes a procedure for making “corrections” to the conventional RTT formulae. The author has formulated these corrections as follows:
Determine the distance to reach reversal floor H, which can be written as
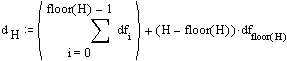 (17)
(17)
(floor(x) is a function which returns the greatest integer less than or equal to x)
The average distance between stops is then

and the flight time to travel this distance is
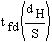
The difference between this and the assumed time can be substituted into an enhanced equation for which becomes
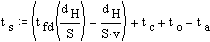 (18)
(18)
Advanced door opening time(s) has also been included in this formulae. Some designers subtract the advanced door opening time from the door opening time, but it is clearer to identify it separately.
The 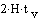 term in the RTT equation also needs to be revised to
term in the RTT equation also needs to be revised to 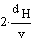 to take into account the new approach. The RTT equation now becomes
to take into account the new approach. The RTT equation now becomes
 (19)
(19)
Equations for  and
and  remain the same.
remain the same.
Express Zones
In high rise buildings lifts are often zoned to reduce passenger travel times and to save core space by not having all the lifts serving the upper floors of the building. An example of a zoned building is represented by the diagram in Figure 1.
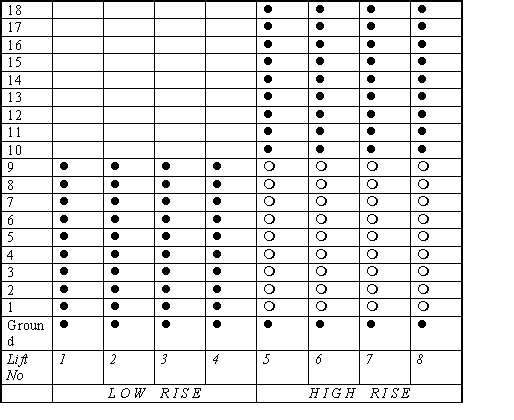
Key  Lift serves floor
Lift serves floor  Lift expresses past floor without stopping
Lift expresses past floor without stopping
Figure 1 Zoned building
This express zone can be taken into account by revising the RTT equation, (19) to
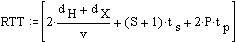 (20)
(20)
where dX is the express zone; in this example, the sum of the floor heights of Levels 1 to 9. It is suggested that equation (20) is used in preference to equation (19) for all cases; if there is no express zone simply set dx to 0.
Discussion
Most lift designs are based on an up peak RTT calculation, which is an important industry standard benchmark. The up peak calculation has been developed over a number of years with contributions from several researchers.
With the improvements discussed in this paper, the up peak calculation can be applied for any combination of floor heights and lift dynamics, including buildings with express zones.
The calculation still has limitations, which include
- only up peak traffic is considered; this is often not the most onerous traffic flow in buildings
- in some instances up peak calculations are inappropriate, e.g. in shopping centres, car parks, airports or hospitals
- it is difficult to adjust the calculation to analyse up peaks for buildings with basements, car parks or more than one entrance floor.
To overcome these limitations, more advanced techniques such as the General Analysis [9][10] and Simulation [11] are required.
Computer software implementing all these analysis techniques is available from the author.
REFERENCES
- Basset Jones The probable number of stops made by an elevator GE Review 26(8) 583-587 (1923)
- Schroeder J Personenaufzeuge Foerden und Heben 1 44-50 (1955) (in German)
- Barney G C, dos Santos S M Elevator Traffic Analysis Design and Control 2nd edn. (London: Peter Peregrinus) (1985)
- Strakosch G R Vertical Transportation: Elevators and Escalators 2nd edn. (New York: J Wiley & Sons Inc.)(1983)
- Peters R D Ideal Lift Kinematics: Complete Equations for Plotting Optimum Motion Elevator Technology 6, Proceedings of ELEVCON’95 (The International Association of Elevator Engineers) (1995) (republished by Elevator World, April 1996 and by Elevatori, May/June 1996)
- Peters R D Ideal Lift Kinematics: Derivation of Formulae for the Equations of Motion of a Lift International Journal of Elevator Engineers, Volume 1 No 1 (1996)
- Motz H D On the kinematics of the ideal motion of lifts Forden und haben 26 (1) (1976) (in German)
- Various Authors CIBSE Guide D, Transportation Systems in Buildings (The Chartered Institution of Building Services Engineers)(1993) ISBN 0 900953 57 8
- Peters R D Lift Traffic Analysis: Formulae for the general case Building Services Engineering Research and Technology, Volume 11 No 2 (1990)
- Peters R D The Theory and Practice of General Analysis Lift Calculations Elevator Technology 4, Proceedings of ELEVCON’92 (The International Association of Elevator Engineers) (1992)
- Peters RD Simulation for control system design and traffic analysis Elevator Technology 9, Proceedings of ELEVCON’98 (The International Association of Elevator Engineers) (1998) (republished by Elevatori, January/February 1999)
